Find trigonometric functions of special angles
key notes :
The cosine (cos) of an angle in a right triangle is a ratio. It is the length of the adjacent leg (adj) divided by the length of the hypotenuse (hyp). The adjacent leg is the leg next to the specified angle and the hypotenuse is the side across from the right angle.

Learn with an example
Evaluate. Write your answer in simplified, rationalised form. Do not round.
cos60° =
To calculate cos60°, draw a right triangle with a 60° angle. This is a 30°-60°-90° triangle, which is a special right triangle. Its side lengths are 1, √3, and 2.

Now, calculate cos60°. The length of the adjacent leg is 1 and the length of the hypotenuse is 2.
cos 60° = adj / hyp Definition of cosine
= 1/2 Plug in adj=1 and hyp=2
So, cos60°= 1/2 .
Evaluate. Write your answer in simplified, rationalised form. Do not round.
sin30° =
To calculate sin30°, draw a right triangle with a 30° angle. This is a 30°-60°-90° triangle, which is a special right triangle. Its side lengths are √3 , 1 and 2.
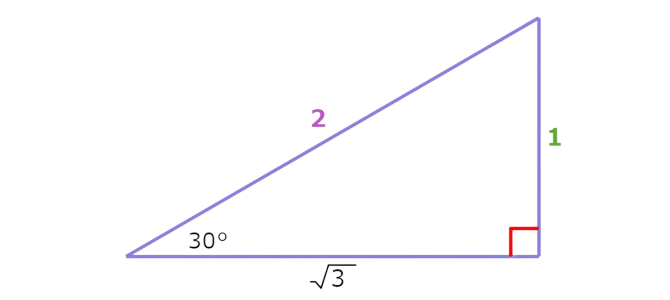
Now, calculate sin30°. The length of the opposite leg is 1 and the length of the hypotenuse is 2.
sin 30° = opp / hyp Definition of sine
= 1/2 Plug in opp=1 and hyp=2
So, sin 30°= 1/2 .
Evaluate. Write your answer in simplified, rationalised form. Do not round.
sin30°=
To calculate sin30°, draw a right triangle with a 30° angle. This is a 30°-60°-90° triangle, which is a special right triangle. Its side lengths are √3 , 1 and 2.

Now, calculate sin30°. The length of the opposite leg is 1 and the length of the hypotenuse is 2.
sin3 0° = opp / hyp Definition of sine
= 1/2 Plug in opp=1 and hyp=2
So, sin 30°= 1/2 .
let’s practice!

